Surface Tension,Cohesion,Adhesive Force,Methods of Measurement

Surface Tension

Surface tension is a contractive tendency of the surface of a liquid that allows it to resist an external force. It is revealed, for example, in the floating of some objects on the surface of water, even though they are denser than water, and in the ability of some insects (e.g. water striders) to run on the water surface. This property is caused by cohesion of similar molecules, and is responsible for many of the behaviors of liquids.

Surface tension has the dimension of force per unit length, or of energy per unit area. The two are equivalent—but when referring to energy per unit of area, people use the term surface energy—which is a more general term in the sense that it applies also to solids and not just liquids.

In materials science, surface tension is used for either surface stress or surface free energy.

The cohesive forces among liquid molecules are responsible for the phenomenon of surface tension. In the bulk of the liquid, each molecule is pulled equally in every direction by neighboring liquid molecules, resulting in a net force of zero. The molecules at the surface do not have other molecules on all sides of them and therefore are pulled inwards. This creates some internal pressure and forces liquid surfaces to contract to the minimal area.

Surface tension is responsible for the shape of liquid droplets. Although easily deformed, droplets of water tend to be pulled into a spherical shape by the cohesive forces of the surface layer. In the absence of other forces, including gravity, drops of virtually all liquids would be perfectly spherical. The spherical shape minimizes the necessary "wall tension" of the surface layer according to Laplace's law.

Another way to view surface tension is in terms of energy. A molecule in contact with a neighbor is in a lower state of energy than if it were alone (not in contact with a neighbor). The interior molecules have as many neighbors as they can possibly have, but the boundary molecules are missing neighbors (compared to interior molecules) and therefore have a higher energy. For the liquid to minimize its energy state, the number of higher energy boundary molecules must be minimized. The minimized quantity of boundary molecules results in a minimized surface area.^[1]

As a result of surface area minimization, a surface will assume the smoothest shape it can (mathematical proof that "smooth" shapes minimize surface area relies on use of the Euler–Lagrange equation). Since any curvature in the surface shape results in greater area, a higher energy will also result. Consequently the surface will push back against any curvature in much the same way as a ball pushed uphill will push back to minimize its gravitational potential energy.

The cohesive forces between liquid molecules are responsible for the phenomenon known as surface tension. The molecules at the surface do not have other like molecules on all sides of them and consequently they cohere more strongly to those directly associated with them on the surface. This forms a surface "film" which makes it more difficult to move an object through the surface than to move it when it is completely submersed.

Surface tension is typically measured in dynes/cm, the force in dynes required to break a film of length 1 cm. Equivalently, it can be stated as surface energy in ergs per square centimeter. Water at 20°C has a surface tension of 72.8 dynes/cm compared to 22.3 for ethyl alcohol and 465 for mercury.

Liquid surface

The reason for this is that the pressure difference across a fluid interface is proportional to the mean curvature, as seen in the Young-Laplace equation. For an open soap film, the pressure difference is zero, hence the mean curvature is zero, and minimal surfaces have the property of zero mean curvature.To find the shape of the minimal surface bounded by some arbitrary shaped frame using strictly mathematical means can be a daunting task. Yet by fashioning the frame out of wire and dipping it in soap-solution, a locally minimal surface will appear in the resulting soap-film within seconds.

Examples of Surface Tension

Walking on water: Small insects such as the water strider can walk on water because their weight is not enough to penetrate the surface.

Floating a needle: A carefully placed small needle can be made to float on the surface of water even though it is several times as dense as water. If the surface is agitated to break up the surface tension, then needle will quickly sink.

Don't touch the tent!: Common tent materials are somewhat rainproof in that the surface tension of water will bridge the pores in the finely woven material. But if you touch the tent material with your finger, you break the surface tension and the rain will drip through.

Clinical test for jaundice: Normal urine has a surface tension of about 66 dynes/centimeter but if bile is present (a test for jaundice), it drops to about 55. In the Hay test, powdered sulfur is sprinkled on the urine surface. It will float on normal urine, but will sink if the surface tension is lowered by the bile.

Surface tension disinfectants: Disinfectants are usually solutions of low surface tension. This allow them to spread out on the cell walls of bacteria and disrupt them.

Soaps and detergents: These help the cleaning of clothes by lowering the surface tension of the water so that it more readily soaks into pores and soiled areas.

Washing with cold water: The major reason for using hot water for washing is that its surface tension is lower and it is a better wetting agent. But if the detergent lowers the surface tension, the heating may be unneccessary.

Why bubbles are round: The surface tension of water provides the necessary wall tension for the formation of bubbles with water. The tendency to minimize that wall tension pulls the bubbles into spherical shapes.

Surface Tension and Droplets: Surface tension is responsible for the shape of liquid droplets. Although easily deformed, droplets of water tend to be pulled into a spherical shape by the cohesive forces of the surface layer.

Cohesion and Surface Tension

The cohesive forces between molecules down into a liquid are shared with all neighboring atoms. Those on the surface have no neighboring atoms above, and exhibit stronger attractive forces upon their nearest neighbors on the surface. This enhancement of the intermolecular attractive forces at the surface is calledsurface tension

Cohesive forces are the intermolecular forces (such as those from hydrogen bonding and Van der Waals forces) which cause a tendency in liquids to resist separation. These attractive forces exist between molecules of the same substance. For instance, rain falls in droplets, rather than a fine mist, because water has strong cohesion which pulls its molecules tightly together, forming droplets. This force tends to unite molecules of a liquid, gathering them into relatively large clusters due to the molecules' dislike for its surrounding..

Adhesive forces are the attractive forces between unlike molecules. They are caused by forces acting between two substances, such as mechanical forces (sticking together) and electrostatic forces (attraction due to opposing charges). In the case of a liquid wetting agent, adhesion causes the liquid to cling to the surface on which it rests. When water is poured on clean glass, it tends to spread, forming a thin, uniform film over the glasses surface. This is because the adhesive forces between water and glass are strong enough to pull the water molecules out of their spherical formation and hold them against the surface of the glass, thus avoiding the repulsion between like molecules.

• When liquid is placed on a smooth surface, the relative strengths of the cohesive and adhesive forces acting on that liquid determine the shape it will take (and whether or not it will wet the surface). If the adhesive forces between a liquid and a surface are stronger, they will pull the liquid down, causing it to wet the surface. However, if they cohesive forces among the liquid itself are stronger, they will resist such adhesion and cause the liquid to retain a spherical shape and bead the surface.

The Meniscus

Meniscus is the curvature of a liquid's surface within a container such as a graduated cylinder. However, before we explain why some liquid have a concave up meniscus while others share a concave down meniscus, first we have to understand the adhesive forces at work on surface tension. 

Water, for example, is a polar molecule that consists of a partial positive charge on the hydrogens and a partial negative charge on the oxygen. Thus, within liquid water, each molecule's partial positive charge is attracted to its neighbor's partial negative charge. This is the origin of the cohesive forces within water. Water molecules buried inside the liquid is then being pulled and pushed evenly in every direction, producing no net pull. Meanwhile, the molecules on the surface of the liquid, lacking pulling forces in the upward direction thus encompass a net downward pull.

Two definitions

Surface tension, represented by the symbol γ is defined as the force along a line of unit length, where the force is parallel to the surface but perpendicular to the line. One way to picture this is to imagine a flat soap film bounded on one side by a taut thread of length, L. The thread will be pulled toward the interior of the film by a force equal to 2L(the factor of 2 is because the soap film has two sides, hence two surfaces).

Surface tension is therefore measured in forces per unit length. Its SI unit is newton per meter but the cgs unit of dyne per cm is also used. One dyn/cm corresponds to 0.001 N/m.

An equivalent definition, one that is useful in thermodynamics, is work done per unit area. As such, in order to increase the surface area of a mass of liquid by an amount, δA, a quantity of work, δA, is needed.This work is stored as potential energy. Consequently surface tension can be also measured in SI system as joules per square meter and in the cgs system as ergs per cm². Since mechanical systems try to find a state of minimum potential energy, a free droplet of liquid naturally assumes a spherical shape, which has the minimum surface area for a given volume.

The equivalence of measurement of energy per unit area to force per unit length can be proven by dimensional analysis.

Surface curvature and pressure

Surface tension forces acting on a tiny (differential) patch of surface. δθ_xand δθ_y indicate the amount of bend over the dimensions of the patch. Balancing the tension forces with pressure leads to the Young–Laplace equation

If no force acts normal to a tensioned surface, the surface must remain flat. But if the pressure on one side of the surface differs from pressure on the other side, the pressure difference times surface area results in a normal force. In order for the surface tension forces to cancel the force due to pressure, the surface must be curved. The diagram shows how surface curvature of a tiny patch of surface leads to a net component of surface tension forces acting normal to the center of the patch. When all the forces are balanced, the resulting equation is known as the Young–Laplace equation:

where:

• Δp is the pressure difference.
•  is surface tension.
• R_x and R_y are radii of curvature in each of the axes that are parallel to the surface.

The quantity in parentheses on the right hand side is in fact (twice) the mean curvature of the surface (depending on normalisation).

Solutions to this equation determine the shape of water drops, puddles, menisci, soap bubbles, and all other shapes determined by surface tension (such as the shape of the impressions that a water strider's feet make on the surface of a pond).

The table below shows how the internal pressure of a water droplet increases with decreasing radius. For not very small drops the effect is subtle, but the pressure difference becomes enormous when the drop sizes approach the molecular size. (In the limit of a single molecule the concept becomes meaningless.)

Methods of measurement

Because surface tension manifests itself in various effects, it offers a number of paths to its measurement. Which method is optimal depends upon the nature of the liquid being measured, the conditions under which its tension is to be measured, and the stability of its surface when it is deformed.

• Du Noüy Ring method: The traditional method used to measure surface or interfacial tension. Wetting properties of the surface or interface have little influence on this measuring technique. Maximum pull exerted on the ring by the surface is measured.Because surface tension manifests itself in various effects, it offers a number of paths to its measurement. Which method is optimal depends upon the nature of the liquid being measured, the conditions under which its tension is to be measured, and the stability of its surface when it is deformed.
• Du Noüy-Padday method: A minimized version of Du Noüy method uses a small diameter metal needle instead of a ring, in combination with a high sensitivity microbalance to record maximum pull. The advantage of this method is that very small sample volumes (down to few tens of microliters) can be measured with very high precision, without the need to correct forbuoyancy (for a needle or rather, rod, with proper geometry). Further, the measurement can be performed very quickly, minimally in about 20 seconds. First commercial multichannel tensiometers  were recently built based on this principle.

• Wilhelmy plate method: A universal method especially suited to check surface tension over long time intervals. A vertical plate of known perimeter is attached to a balance, and the force due to wetting is measured.

• Spinning drop method: This technique is ideal for measuring low interfacial tensions. The diameter of a drop within a heavy phase is measured while both are rotated.

• Pendant drop method: Surface and interfacial tension can be measured by this technique, even at elevated temperatures and pressures. Geometry of a drop is analyzed optically. For details, see Drop.

• Bubble pressure method (Jaeger's method): A measurement technique for determining surface tension at short surface ages. Maximum pressure of each bubble is measured.

• Drop volume method: A method for determining interfacial tension as a function of interface age. Liquid of one density is pumped into a second liquid of a different density and time between drops produced is measured.

• Capillary rise method: The end of a capillary is immersed into the solution. The height at which the solution reaches inside the capillary is related to the surface tension by the equation discussed below.

• Stalagmometric method: A method of weighting and reading a drop of liquid.

• Sessile drop method: A method for determining surface tension and density by placing a drop on a substrate and measuring the contact angle (see Sessile drop technique).

• Vibrational frequency of levitated drops: The natural frequency of vibrational oscillations of magnetically levitated drops has been used to measure the surface tension of superfluid⁴He. This value is estimated to be 0.375 dyn/cm at T = 0 K.

Liquid in a vertical tube

Diagram of a mercury barometer

An old style mercury barometer consists of a vertical glass tube about 1 cm in diameter partially filled with mercury, and with a vacuum (calledTorricelli's vacuum) in the unfilled volume (see diagram to the right). Notice that the mercury level at the center of the tube is higher than at the edges, making the upper surface of the mercury dome-shaped. The center of mass of the entire column of mercury would be slightly lower if the top surface of the mercury were flat over the entire crossection of the tube. But the dome-shaped top gives slightly less surface area to the entire mass of mercury. Again the two effects combine to minimize the total potential energy. Such a surface shape is known as a convex meniscus.

The reason we consider the surface area of the entire mass of mercury, including the part of the surface that is in contact with the glass, is because mercury does not adhere at all to glass. So the surface tension of the mercury acts over its entire surface area, including where it is in contact with the glass. If instead of glass, the tube were made out of copper, the situation would be very different. Mercury aggressively adheres to copper. So in a copper tube, the level of mercury at the center of the tube will be lower than at the edges (that is, it would be a concave meniscus). In a situation where the liquid adheres to the walls of its container, we consider the part of the fluid's surface area that is in contact with the container to have negativesurface tension. The fluid then works to maximize the contact surface area. So in this case increasing the area in contact with the container decreases rather than increases the potential energy. That decrease is enough to compensate for the increased potential energy associated with lifting the fluid near the walls of the container.

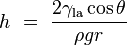
If a tube is sufficiently narrow and the liquid adhesion to its walls is sufficiently strong, surface tension can draw liquid up the tube in a phenomenon known as capillary action. The height the column is lifted to is given by:

where

•  is the height the liquid is lifted,
•  is the liquid-air surface tension,
•  is the density of the liquid,
•  is the radius of the capillary,
•  is the acceleration due to gravity,
•  is the angle of contact described above. If  is greater than 90°, as with mercury in a glass container, the liquid will be depressed rather than lifted.

Capillary attraction

Capillary attraction, or capillarity, is the ability of a liquid to flow in narrow spaces without the assistance of, and in opposition to external forces like gravity. The effect can be seen in the drawing up of liquids between the hairs of a paint-brush, in a thin tube, in porous materials such as paper, in some non-porous materials such as liquified carbon fiber, or in a cell. It occurs because of inter-molecular attractive forces between the liquid and solid surrounding surfaces. If the diameter of the tube is sufficiently small, then the combination of surface tension(which is caused by cohesion within the liquid) and adhesive forces between the liquid and container act to lift the liquid.

Capillary action, capillarity, capillary motion, or wicking refers to two phenomena:

A common apparatus used to demonstrate the first phenomenon is the capillary tube. When the lower end of a vertical glass tube is placed in a liquid such as water, a concave meniscus forms. Adhesion forces between the fluid and the solid inner wall pulls the liquid column up until there is a sufficient mass of liquid for gravitational forces to overcome these intermolecular forces. The contact length (around the edge) between the top of the liquid column and the tube is proportional to the diameter of the tube, while the weight of the liquid column is proportional to the square of the tube's diameter, so a narrow tube will draw a liquid column higher than a wide tube.

Yüzey Gerilimi,Etkiyen Kuvvetler ve Bulunması İçin Gerekli Metotlar

Yüzey Gerilim Kuvvetleri


 Gazlarda söz konusu olmayan yüzey gerilimi sıvı ve katılara özgü bir olgudur.Kütlesel çekim kuvvetinin olmadığı bir yere örneğin uzay boşluğuna bırakılan bir miktar sıvı küre şeklini alarak hemen en küçük yüzey alanına sahip olur.
 Sıvının içindeki moleküller üzerine etkiyen çekim kuvvetlerinin bileşkesi sıfır olduğu halde sıvı yüzeyindeki moleküller sıvı içine doğru çeken net bir kuvvetin etkisi altındadır.Yüzeyi küçültmeye çalışan bu kuvvetleri yenmek için dışarıdan sıvıya enerji vermek gerekmektedir. Sabit sıcaklık ve basınçta sıvı yüzeyini 1m^2 veya 1cm^2 büyütmek için verilmesi gereken enerjiye yüzey gerilimi adı verilir.

 Bütün sıvılarda şiddeti sıvının türüne göre değişen moleküller arası çekim kuvvetleri (kohezyon kuvvetleri) bulunmaktadır. Sıvılarda iç kısımlarda (sıvının çeşitli derinliklerinde bulunan) moleküller çevresindeki komşu moleküller tarafından her yönden eşit olarak , diğer bir ifadeyle küresel simetrik şekilde, çekim kuvvetlerinin etkisi altında bulunurlar. Böylece sıvı içerisindeki bir moleküle etkiyen kuvvetler birbirlerini dengeler. Oysa sıvının yüzeyinde bulunan bir molekül (sıvı- buhar ara yüzeyi göz önüne alındığında) buhar fazındaki yoğunluk sıvı fazdan düşük olduğundan, sadece yüzeyin altındaki moleküller tarafından sıvının içerisine doğru çekilirler. Sıvı içerisindeki moleküller, yüzeydekilere göre daha fazla çekim kuvvetinin etkisi altında bulunduklarından potansiyel enerjileri, yüzeydeki moleküllerin potansiyel enerjilerinden daha düşüktür. Çünkü genel olarak bilinmektedir ki bir cisme etki eden çekim kuvvetleri ne kadar fazla ise cismin potansiyel enerjisi o kadar düşüktür.

Sıvını iç kısmındaki molekülleri yüzeye çıkararak sıvının serbest yüzeyini artırmak için, sıvı molekülleri arasındaki kohezyon kuvvetlerine karşı iş yapılmalıdır. Bunun sonucu olarak sıvının yüzey bölgesinin molar serbest enerjisi , sıvının diğer kısmının molar serbest enerjisinden yüksektir. 1805 Thomas Young sıvı yüzeyinin mekanik özelliklerinin, yüzey üzerine gerilmiş hayali bir zarın mekanik özellikleri ile ilişkilendirilebileceğini gösterdi. Böylece sıvı yüzeyi moleküller arasında mevcut olan kohezyon kuvvetlerinin sonucu olarak , bir bakımdan gerilmiş hayali bir zar gibi daima büzülmek isteyen ve mümkün olan en küçük yüzeyi almak isteyen 1molekül kalınlığında çok ince zar gibi düşünülebilir.

Bir sıvının yüzey gerilimi (g ); yüzey üzerinde sıvının yüzey genişlemesine zıt olan birim uzunluk başına kuvvetdir. Yüzey gerilimi, yüzeye paralel olarak etkir. Yüzey geriliminin SI sistemindeki birimi metre başına Newton (Nm-1) veya (1J=Nm olduğundan) Jm-2 dir. CGS sistemindeki birimi ise dyn/cm yada erg/cm2 dir. Örneğin suyun yüzey gerilimi 20 0C de 72.8 dyn/cm veya 72.8 erg/cm2 olduğundan suyun yüzeyini 20 0C de 1cm2 genişletebilmek için 72.8 erglik bir enerjiye veya 1cm boyunca sıvı yüzeyinde yer alan moleküller arası ilişkileri kesebilmek için 72.8 dyn lik bir kuvvete ihtiyaç var demektir.

Yüzey geriliminin sebebi nedir?

Bu kuvvetin kaynağı temel olarak su moleküllerini bir arada tutan moleküller arası çekici kuvvetlerdir. Suyun içinde olan moleküller her yönden komşu moleküllerle kuşatıldıkları için, üzerlerine etkiyen toplam kuvvet sıfırdır. Buna karşın, yüzeydeki moleküllerin sadece bir tarafı diğer su molekülleriyle çevrili olduğu için, bunlar içeriye doğru net bir kuvvetle çekilirler. Bu durum yüzeyde bir gerilme oluşturup yüzeyin minimum olmasını sağlar. Hacimleri eşit birçok geometrik şekil içinde yüzey alanı en az olan küredir. Su damlalarının küresel bir şekil alması da yüzey geriliminin en az yüzey oluşturacak şekilde molekülleri hareket ettirmesidir.



Yüzey Gerilimi nasıl değişir?



Sıvı üzerindeki gaz yoğunluğu çok fazla arttırıldığında veya bu sıvı üzerine bu sıvıda çözünmeyen bir başka sıvı ilave edildiğinde sıvının yüzey gerilimi karşı fazdaki moleküllerle gireceği moleküler etkileşmeler sonucu bir miktar azalacaktır.

Çoğu sıvıların yüzey gerilimleri artan sıcaklıkla doğrusal bir şekilde azalır(bazı erimiş metaller hariç ) ve moleküller arası kohezyon kuvvetlerinin sıfıra yaklaştığı kritik sıcaklık civarında çok küçük bir değer olur.

Saf bir madde içerisinde bir madde çözünüyorsa çözünen maddenin ve çözücünün karakterine bağlı olarak yüzey geriliminin değiştiği gözlenmiştir. Ayrıca yapılan incelemelerle çözünen maddenin sıvının iç kısımlarındaki konsantrasyonun birbirinden farklı olduğu gözlenmiştir ki bu beklenen bir olaydır.




Sıvı - Sıvı Arayüzey Gerilimi


Birbiri içinde çözünmeyen iki sıvı ele alalım. Bunların birbirlerine temas noktasında bir yüzey gerilimi vardır ve bu nokta ne üstteki ne de alttaki sıvıya benzemektedir. Bu sıvıların her birinin ayrı ayrı yüzey gerilimleri toplamı bu iki sıvının oluşturduğu ara yüzey geriliminden her zaman büyüktür.

Her sıvının yüzey geriliminde bir azalma olacağına göre bir başka deyişle sıvılar yüzey serbest enerjilerini azalttıklarına göre bu sıvıları birbirinden ayırabilmek için bir iş yapmak gerekir. Sıvılar farklı ise bu işe adezyon işi denir. Ve aşağıdaki gibi hesaplanır;

Bu iş sıvılar aynı ise kohezyon işi olarak adlandırılır.

Aslında kohezyon işi, bir sıvıyı ikiye bölüp yeni bir yüzey oluşturulabilmek için verilmesi gereken enerji miktarıdır.




Çözeltilerin Yüzey Gerilimi

Çözünen tanecikler içteki çözücü moleküllerinin yüzeydeki çözücü moleküllerini içe doğru çekmesini belli ölçüde engellediğinden çözeltilerin yüzey gerilimi saf çözücüye göre genellikle düşüktür. Çözücünün yüzey gerilimini düşüren maddeler yüzey aktif, değiştirmeyenler ise yüzey inaktif olarak isimlendirilmektedir. Sulu çözeltiler için yüzey aktif maddeleri; organik asitler,alkoller, esterler, eterler,aminler ve ketonlar şeklinde; yüzey inaktif maddeleri ise inorganik elektrolitler,organik asitlerin tuzları,molar kütleleri küçük olan bazlar yanında şeker ve gliserin gibi uçucu ve elektrolit olmayan maddeler şeklinde sıralayabiliriz.

Yağ asitleri gibi suyun yüzey gerilimini önemli ölçüde düşüren maddeler, hem polar hidrofilik(su seven)grup hem de apolar hidrofobik (su sevmeyen)grup ihtiva ederler. Yağ asitlerindeki –COOH grubu gibi hidrofilik gruplar, eğer molekülün kalan apolar kısmı çok büyük değilse, molekülün çözünürlüğünü arttırır. Yağ asitlerinin hidrokarbon kısımları bir sulu çözeltinin iç kısımlarında rahatsızlık duyarlar (yani yüksek bir serbest enerjiye sahiptirler) ve onlar sıvının iç kısmından yüzeye getirmek çok az iş gerektirir. Bu sebeple yüzey gerilimini düşüren bir çözünen(yüzey aktif madde), çözeltinin yüzey tabakalarında birikir. Böyle çözünenlerin ara yüzeyde “pozitif adsorblandığı” söylenir.


Yüzey Geriliminin Ölçülmesi

Yüzey gerilimi ölçümü için birbirinden farklı çok sayıda yöntem vardır.
Sıvıların kılcalda yükselmesi veya düşmesi,damla kütlelerini belirleme veya damla sayma,bir halkanın sıvıdan koparılması ve maksimum kabarcık basıncının belirlenmesi bunlardan en önemli olanlarıdır.

 Su gibi ıslatan sıvıların molekülleri ile cam arasındaki çekim kuvvetleri sıvı moleküllerinin birbiri arasındaki çekim kuvvetlerinden daha büyüktür.Sıvı ile cam arasındaki çekim kuvvetlerine adezyon kuvvetleri denir. Kılcal boruda yükselmeye bu adezyon kuvvetleri yol açmaktadır. Civa gibi ıslatmayan sıvının molekülleri ile cam arasıdaki çekim kuvvetleri sıvı moleküllerin birbiri arasındaki çekim kuvvetlerinden çok küçük kalmaktadır.
Sıvı molekülleri arasındaki çekim kuvvetlerine kohezyon kuvvetleri adı verilir.Kılcal boruda alçalmaya yol açan bu kuvvetler kohezyon kuvvetleridir.
Adezyon kuvvetinin etkin olduğu kılcallarda sıvı yüzeyi iç bükey,kohezyon kuvvetinin etkin oldugu kılcallarda ise dış bükeydir.Kılcal olmayan borularda sıvı yüzeyi düz görünür.

Yüzey gerilimi ile ilgili bazı özellikler;

• Sıcaklığı artan bir maddenin yüzey gerilimi azalır.
• İyonik tuzların suda çözülmesi yüzey gerilimini arttırır.
• Yüzey gerilimi sayesinde böcekler su yüzeyinde yürüyebilir.
• Her sıvının kendine ait bir yüzey gerilimi katsayısı vardır, ayırt edici özelliktir.

Viscosity and Effecting Factors

Viscosity is a measure of the resistance of a fluid which is being deformed by eithershear stress or tensile stress. In everyday terms (and for fluids only), viscosity is "thickness" or "internal friction". Thus, water is "thin", having a lower viscosity, whilehoney is "thick", having a higher viscosity. Put simply, the less viscous the fluid is, the greater its ease of movement (fluidity)

Viscosity describes a fluid's internal resistance to flow and may be thought of as a measure of fluid friction. For example, high-viscosity felsic magma will create a tall, steep stratovolcano, because it cannot flow far before it cools, while low-viscositymafic lava will create a wide, shallow-sloped shield volcano.

With the exception of superfluids, all real fluids have some resistance to stress and therefore are viscous. A fluid which has no resistance to shear stress is known as anideal fluid or inviscid fluid. In common usage, a liquid with the viscosity less than water is known as a mobile liquid, while a substance with a viscosity substantially greater than water is simply called a viscous liquid.

The SI unit of viscosity is the pascal second [Pa s], which has no special name. Despite its self-proclaimed title as an international system, the International System of Units has had very little international impact on viscosity. The pascal second is rarely used in scientific and technical publications today. The most common unit of viscosity is the dyne second per square centimeter[dyne s/cm²], which is given the name poise [P] after the French physiologist Jean Louis Poiseuille(1799-1869). Ten poise equal one pascal second [Pa s] making the centipoise [cP] andmillipascal second [mPa s] identical.

1 pascal second = 10 poise = 1,000 millipascal second
1 centipoise = 1 millipascal second

There are actually two quantities that are called viscosity. The quantity defined above is sometimes called dynamic viscosity, absolute viscosity, or simple viscosity to distinguish it from the other quantity, but is usually just called viscosity. The other quantity called kinematic viscosity (represented by the symbol ν "nu") is the ratio of the viscosity of a fluid to its density.

                                                  ν = η/ρ

Kinematic viscosity is a measure of the resistive flow of a fluid under the influence of gravity. It is frequently measured using a device called a capillary viscometer — basically a graduated can with a narrow tube at the bottom. When two fluids of equal volume are placed in identical capillary viscometers and allowed to flow under the influence of gravity, a viscous fluid takes longer than a less viscous fluid to flow through the tube. Capillary viscometers are discussed in more detail later in this section.

The SI unit of kinematic viscosity is the square meter per second [m²/s], which has no special name. This unit is so large that it is rarely used. A more common unit of kinematic viscosity is thesquare centimeter per second [cm²/s], which is given the name stokes [St] after the Irish mathematician and physicist George Gabriel Stokes (1819-1903). Even this unit is also a bit too large and so the most common unit is probably the square millimeter per second [mm²/s] orcentistokes [cSt].

1 m²/s = 10,000 cm²/s [stokes] = 1,000,000 mm²/s [centistokes]
1 cm²/s = 1 stokes
1 mm²/s = 1 centistokes

Factors Affecting Viscosity 

Viscosity is first and foremost a function of material. The viscosity of water at 20 ℃ is 1.0020 millipascal seconds (which is conveniently close to one by coincidence alone). Most ordinary liquids have viscosities on the order of 1 to 1000 mPa s, while gases have viscosities on the order of 1 to 10 μPa s. Pastes, gels, emulsions, and other complex liquids are harder to summarize. Some fats like butter or margarine are so viscous that they seem more like soft solids than like flowing liquids. Molten glass is extremely viscous and approaches infinite viscosity as it solidifies. Since this process is not as well defined as true freezing, some believe (incorrectly) that glass may still flow even after it has completely cooled, but this is not the case. At ordinary temperatures, glasses are as solid as true solids.

From everyday experience, it should be common knowledge that viscosity varies with temperature. Honey and syrups can be made to flow more readily when heated. Engine oil and hydraulic fluids thicken appreciably on cold days and significantly affect the performance of cars and other machinery during the winter months. In general, the viscosity of a simple liquiddecreases with increasing temperature (and vice versa). As temperature increases, the average speed of the molecules in a liquid increases and the amount of time they spend "in contact" with their nearest neighbors decreases. Thus, as temperature increases, the average intermolecular forces decrease. The exact manner in which the two quantities vary is nonlinear and changes abruptly when the liquid changes phase.

Viscosity is normally independent of pressure, but liquids under extreme pressure often experience an increase in viscosity. Since liquids are normally incompressible, an increase in pressure doesn't really bring the molecules significantly closer together. Simple models of molecular interactions won't work to explain this behavior and, to my knowledge, there is no generally accepted more complex model that does. The liquid phase is probably the least well understood of all the phases of matter.

While liquids get runnier as they get hotter, gases get thicker. (If one can imagine a "thick" gas.) The viscosity of gases increases as temperature increases and is approximately proportional to the square root of temperature. This is due to the increase in the frequency of intermolecular collisions at higher temperatures. Since most of the time the molecules in a gas are flying freely through the void, anything that increases the number of times one molecule is in contact with another will decrease the ability of the molecules as a whole to engage in the coordinated movement. The more these molecules collide with one another, the more disorganized their motion becomes. Physical models, advanced beyond the scope of this book, have been around for nearly a century that adequately explain the temperature dependence of viscosity in gases. Newer models do a better job than the older models. They also agree with the observation that the viscosity of gases is roughly independent of pressure and density. The gaseous phase is probably the best understood of all the phases of matter.

Since viscosity is so dependent on temperature, it shouldn't never be stated without it.

How does temperature effect viscosity of liquids?

-As the temperature of the liquid fluid increases its viscosity decreases. In the liquids the cohesive forces between the molecules predominates the molecular momentum transfer between the molecules, mainly because the molecules are closely packed (it is this reason that liquids have lesser volume than gases. 

-The viscosity of the gases increases as the temperature of the gas increases. The reason behind this is again the movement of the molecules and the forces between them. In the gases the cohesive forces between the molecules is lesser, while molecular momentum transfer is high. As the temperature of the gas is increased the molecular momentum transfer rate increases further which increases the viscosity of the gas.

CApillary viscometer

The the mathematical expression describing the flow of fluids in circular tubes was determined by the French physician and physiologist Jean Louis Marie Poiseuille (1799–1869). Since it was also discovered independently by the German hydraulic engineer Gotthilf Hagen (1797–1884), it should be properly known as the Hagen-Poiseuille equation, but it is usually just calledPoiseuille's equation. I will not derive it here. (Please don't ask me to.) For non-turbulent, non-pulsatile fluid flow through a uniform straight pipe, the volume flow rate (φ) is …

• directly proportional to the pressure difference (ΔP) between the ends of the tube,
• inversely proportional to the length (ℓ) of the tube,
• inversely proportional to the viscosity (η) of the fluid, and
• proportional to the fourth power of the radius (r⁴) of the tube.

                                                   

φ =	πΔPr4
	8ηℓ

falling sphere

The mathematical expression describing the viscous drag force on a sphere was determined by the British physicist George Gabriel Stokes (1819–1903). I will not derive it here. (Once again, don't ask.)

R = 6πηrv

The formula for the buoyant force on a sphere is accredited to the Greek engineer Archimedesa.k.a. Αρχιμήδης (287–212 BCE), but equations weren't invented back then.

B = ρ_fluidgV_displaced

The formula for weight had to be invented by someone, but I don't know who.

W = mg = ρ_objectgV_object

Let's combine all these things together for a sphere falling in a fluid. Weight goes down, buoyancy goes up, drag goes up. After awhile, the sphere will fall with constant velocity. When it does, all these forces cancel. When a sphere is falling through a fluid it is completely submerged, so there is only one volume to talk about — the volume of a sphere. Let's work through this.

B	+	R	=	W
ρfluidgV	+	6πηrv	=	ρobjectgV
		6πηrv	=	(ρobject − ρfluid)gV
		6πηrv	=	Δρg	4	πr3
					3

And here we are.

η =	2Δρgr2
	9v

Drop a sphere into a liquid. If you know the size and density of the sphere and the density of the liquid, you can determine the viscosity of the liquid. If you don't know the density of the fluid you can determine the kinematic viscosity. If you don't know the density of the sphere, but you know its mass and radius, well then you do know its density. Why are you talking to me? Go back several chapters and get yourself some education.

Viscosity of Solids

On the basis that all solids such as granite flow in response to small shear stress, some researchers have contended that substances known as amorphous solids, such as glass and many polymers, may be considered to have viscosity. This has led some to the view that solids are simply "liquids" with a very high viscosity, typically greater than 10¹² Pa·s. This position is often adopted by supporters of the widely held misconception that glass flow can be observed in old buildings. This distortion is the result of the undeveloped glass making process of earlier eras, and not due to the viscosity of glass.

However, others argue that solids are, in general, elastic for small stresses while fluids are not. Even if solids flow at higher stresses, they are characterized by their low-stress behavior. This distinction is muddled if measurements are continued over long time periods, such as the pitch drop experiment. Viscosity may be an appropriate characteristic for solids in a plastic regime. The situation becomes somewhat confused as the term viscosity is sometimes used for solid materials, for example Maxwell materials, to describe the relationship between stress and the rate of change of strain, rather than rate of shear.

These distinctions may be largely resolved by considering the constitutive equations of the material in question, which take into account both its viscous and elastic behaviors. Materials for which both their viscosity and their elasticity are important in a particular range of deformation and deformation rate are called viscoelastic. In geology, earth materials that exhibit viscous deformation at least three times greater than their elastic deformation are sometimes called rheids.